g0901_1000.s0998_maximum_binary_tree_ii.readme.md Maven / Gradle / Ivy
Go to download
Show more of this group Show more artifacts with this name
Show all versions of leetcode-in-java Show documentation
Show all versions of leetcode-in-java Show documentation
Java-based LeetCode algorithm problem solutions, regularly updated
998\. Maximum Binary Tree II
Medium
A **maximum tree** is a tree where every node has a value greater than any other value in its subtree.
You are given the `root` of a maximum binary tree and an integer `val`.
Just as in the [previous problem](https://leetcode.com/problems/maximum-binary-tree/), the given tree was constructed from a list `a` (`root = Construct(a)`) recursively with the following `Construct(a)` routine:
* If `a` is empty, return `null`.
* Otherwise, let `a[i]` be the largest element of `a`. Create a `root` node with the value `a[i]`.
* The left child of `root` will be `Construct([a[0], a[1], ..., a[i - 1]])`.
* The right child of `root` will be `Construct([a[i + 1], a[i + 2], ..., a[a.length - 1]])`.
* Return `root`.
Note that we were not given `a` directly, only a root node `root = Construct(a)`.
Suppose `b` is a copy of `a` with the value `val` appended to it. It is guaranteed that `b` has unique values.
Return `Construct(b)`.
**Example 1:**
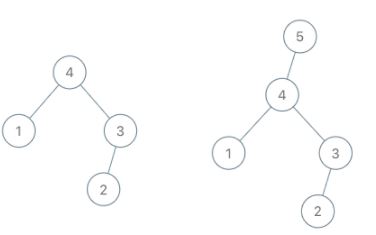
**Input:** root = [4,1,3,null,null,2], val = 5
**Output:** [5,4,null,1,3,null,null,2]
**Explanation:** a = [1,4,2,3], b = [1,4,2,3,5]
**Example 2:**
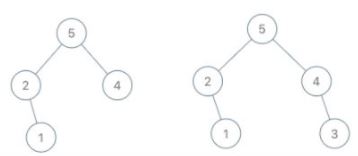
**Input:** root = [5,2,4,null,1], val = 3
**Output:** [5,2,4,null,1,null,3]
**Explanation:** a = [2,1,5,4], b = [2,1,5,4,3]
**Example 3:**

**Input:** root = [5,2,3,null,1], val = 4
**Output:** [5,2,4,null,1,3]
**Explanation:** a = [2,1,5,3], b = [2,1,5,3,4]
**Constraints:**
* The number of nodes in the tree is in the range `[1, 100]`.
* `1 <= Node.val <= 100`
* All the values of the tree are **unique**.
* `1 <= val <= 100`