g0101_0200.s0173_binary_search_tree_iterator.readme.md Maven / Gradle / Ivy

Go to download

Show more of this group Show more artifacts with this name
Show all versions of leetcode-in-java Show documentation

Java-based LeetCode algorithm problem solutions, regularly updated

The newest version!

173\. Binary Search Tree Iterator

Medium

Implement the `BSTIterator` class that represents an iterator over the **[in-order traversal](https://en.wikipedia.org/wiki/Tree_traversal#In-order_(LNR))** of a binary search tree (BST):

*   `BSTIterator(TreeNode root)` Initializes an object of the `BSTIterator` class. The `root` of the BST is given as part of the constructor. The pointer should be initialized to a non-existent number smaller than any element in the BST.
*   `boolean hasNext()` Returns `true` if there exists a number in the traversal to the right of the pointer, otherwise returns `false`.
*   `int next()` Moves the pointer to the right, then returns the number at the pointer.

Notice that by initializing the pointer to a non-existent smallest number, the first call to `next()` will return the smallest element in the BST.

You may assume that `next()` calls will always be valid. That is, there will be at least a next number in the in-order traversal when `next()` is called.

**Example 1:**

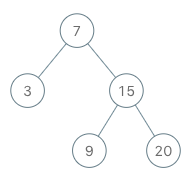
![](https://assets.leetcode.com/uploads/2018/12/25/bst-tree.png)

**Input** ["BSTIterator", "next", "next", "hasNext", "next", "hasNext", "next", "hasNext", "next", "hasNext"] [[[7, 3, 15, null, null, 9, 20]], [], [], [], [], [], [], [], [], []]

**Output:** [null, 3, 7, true, 9, true, 15, true, 20, false]

**Explanation:**

    BSTIterator bSTIterator = new BSTIterator([7, 3, 15, null, null, 9, 20]);
    bSTIterator.next(); // return 3
    bSTIterator.next(); // return 7
    bSTIterator.hasNext(); // return True
    bSTIterator.next(); // return 9
    bSTIterator.hasNext(); // return True
    bSTIterator.next(); // return 15
    bSTIterator.hasNext(); // return True
    bSTIterator.next(); // return 20
    bSTIterator.hasNext(); // return False 

**Constraints:**

*   The number of nodes in the tree is in the range [1, 10⁵].
*   0 <= Node.val <= 10⁶
*   At most 10⁵ calls will be made to `hasNext`, and `next`.

**Follow up:**

*   Could you implement `next()` and `hasNext()` to run in average `O(1)` time and use `O(h)` memory, where `h` is the height of the tree?