• Home
• com.github.javadev
• leetcode-in-java17
• 1.9
• source code
• readme.md

×

Project download

Many resources are needed to download a project. Please understand that we have to compensate our server costs. Thank you in advance.
Project price only 1 $

You can buy this project and download/modify it how often you want.

g1501_1600.s1514_path_with_maximum_probability.readme.md Maven / Gradle / Ivy

1514\. Path with Maximum Probability

Medium

You are given an undirected weighted graph of `n` nodes (0-indexed), represented by an edge list where `edges[i] = [a, b]` is an undirected edge connecting the nodes `a` and `b` with a probability of success of traversing that edge `succProb[i]`.

Given two nodes `start` and `end`, find the path with the maximum probability of success to go from `start` to `end` and return its success probability.

If there is no path from `start` to `end`, **return 0**. Your answer will be accepted if it differs from the correct answer by at most **1e-5**.

**Example 1:**

**![](https://assets.leetcode.com/uploads/2019/09/20/1558_ex1.png)**

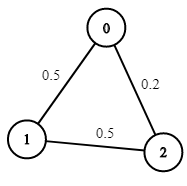**Input:** n = 3, edges = [[0,1],[1,2],[0,2]], succProb = [0.5,0.5,0.2], start = 0, end = 2

**Output:** 0.25000

**Explanation:** There are two paths from start to end, one having a probability of success = 0.2 and the other has 0.5 \* 0.5 = 0.25.

**Example 2:**

**![](https://assets.leetcode.com/uploads/2019/09/20/1558_ex2.png)**

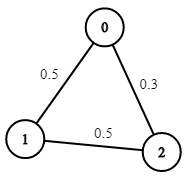**Input:** n = 3, edges = [[0,1],[1,2],[0,2]], succProb = [0.5,0.5,0.3], start = 0, end = 2

**Output:** 0.30000

**Example 3:**

**![](https://assets.leetcode.com/uploads/2019/09/20/1558_ex3.png)**

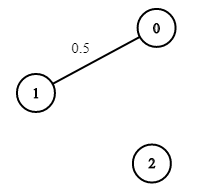**Input:** n = 3, edges = [[0,1]], succProb = [0.5], start = 0, end = 2

**Output:** 0.00000

**Explanation:** There is no path between 0 and 2.

**Constraints:**

*   `2 <= n <= 10^4`
*   `0 <= start, end < n`
*   `start != end`
*   `0 <= a, b < n`
*   `a != b`
*   `0 <= succProb.length == edges.length <= 2*10^4`
*   `0 <= succProb[i] <= 1`
*   There is at most one edge between every two nodes.