
g0201_0300.s0221_maximal_square.Solution Maven / Gradle / Ivy
Go to download
Show more of this group Show more artifacts with this name
Show all versions of leetcode-in-java21 Show documentation
Show all versions of leetcode-in-java21 Show documentation
Java-based LeetCode algorithm problem solutions, regularly updated
package g0201_0300.s0221_maximal_square;
// #Medium #Top_100_Liked_Questions #Array #Dynamic_Programming #Matrix
// #Dynamic_Programming_I_Day_16 #Big_O_Time_O(m*n)_Space_O(m*n)
// #2022_07_04_Time_7_ms_(72.35%)_Space_59.5_MB_(10.55%)
/**
* 221 - Maximal Square\.
*
* Medium
*
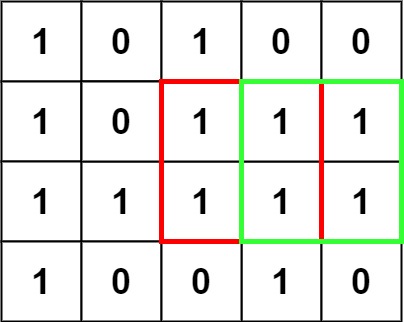
* Given an `m x n` binary `matrix` filled with `0`'s and `1`'s, _find the largest square containing only_ `1`'s _and return its area_.
*
* **Example 1:**
*
* 
*
* **Input:** matrix = \[\["1","0","1","0","0"],["1","0","1","1","1"],["1","1","1","1","1"],["1","0","0","1","0"]]
*
* **Output:** 4
*
* **Example 2:**
*
* 
*
* **Input:** matrix = \[\["0","1"],["1","0"]]
*
* **Output:** 1
*
* **Example 3:**
*
* **Input:** matrix = \[\["0"]]
*
* **Output:** 0
*
* **Constraints:**
*
* * `m == matrix.length`
* * `n == matrix[i].length`
* * `1 <= m, n <= 300`
* * `matrix[i][j]` is `'0'` or `'1'`.
**/
public class Solution {
public int maximalSquare(char[][] matrix) {
int m = matrix.length;
if (m == 0) {
return 0;
}
int n = matrix[0].length;
if (n == 0) {
return 0;
}
int[][] dp = new int[m + 1][n + 1];
int max = 0;
for (int i = 0; i < m; i++) {
for (int j = 0; j < n; j++) {
if (matrix[i][j] == '1') {
// 1 + minimum from cell above, cell to the left, cell diagonal upper-left
int next = 1 + Math.min(dp[i][j], Math.min(dp[i + 1][j], dp[i][j + 1]));
// keep track of the maximum value seen
if (next > max) {
max = next;
}
dp[i + 1][j + 1] = next;
}
}
}
return max * max;
}
}